Leetcode 1631. Path With Minimum Effort

You are a hiker preparing for an upcoming hike. You are given heights, a 2D array of size rows x columns, where heights[row][col] represents the height of cell (row, col). You are situated in the top-left cell, (0, 0), and you hope to travel to the bottom-right cell, (rows-1, columns-1) (i.e., 0-indexed). You can move up, down, left, or right, and you wish to find a route that requires the minimum effort.

A route’s effort is the maximum absolute difference in heights between two consecutive cells of the route.

Return the minimum effort required to travel from the top-left cell to the bottom-right cell.

Example 1:

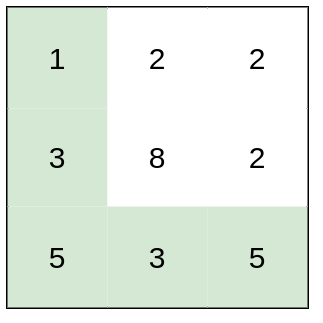
Input: heights = [[1,2,2],[3,8,2],[5,3,5]] Output: 2 Explanation: The route of [1,3,5,3,5] has a maximum absolute difference of 2 in consecutive cells. This is better than the route of [1,2,2,2,5], where the maximum absolute difference is 3.

Example 2:

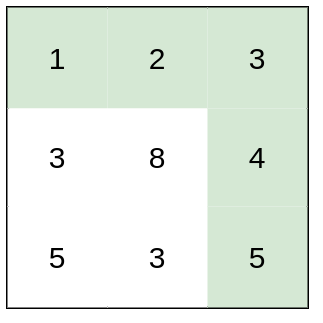
Input: heights = [[1,2,3],[3,8,4],[5,3,5]] Output: 1 Explanation: The route of [1,2,3,4,5] has a maximum absolute difference of 1 in consecutive cells, which is better than route [1,3,5,3,5].

Example 3:

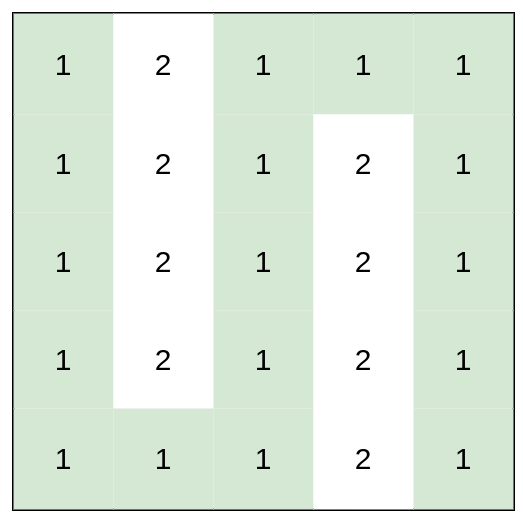
Input: heights = [[1,2,1,1,1],[1,2,1,2,1],[1,2,1,2,1],[1,2,1,2,1],[1,1,1,2,1]] Output: 0 Explanation: This route does not require any effort.

Constraints:

• rows == heights.length
• columns == heights[i].length
• 1 <= rows, columns <= 100
• 1 <= heights[i][j] <= 106

This problem can be solved by using Dijkstra’s algorithm as well, but you may need to think about it a little bit.

class Solution:
    def minimumEffortPath(self, heights: List[List[int]]) -> int:
        visited = set()
        minHeap = [(0, 0, 0)]

        dest = (len(heights)-1, len(heights[0])-1)

        while minHeap:
            max_diff, row, col = heapq.heappop(minHeap)
            
            if (row, col) in visited:
                continue
            
            if row == dest[0] and col == dest[1]:
                return max_diff

            current_height = heights[row][col]

            if row+1 <= dest[0]:
                next_height = heights[row+1][col]
                next_diff = max(max_diff, abs(next_height - current_height))
                heapq.heappush(minHeap, (next_diff, row+1, col))
            
            if row-1 >= 0:
                next_height = heights[row-1][col]
                next_diff = max(max_diff, abs(next_height - current_height))
                heapq.heappush(minHeap, (next_diff, row-1, col))
            
            if col+1 <= dest[1]:
                next_height = heights[row][col+1]
                next_diff = max(max_diff, abs(next_height - current_height))
                heapq.heappush(minHeap, (next_diff, row, col+1))

            if col-1 >= 0:
                next_height = heights[row][col-1]
                next_diff = max(max_diff, abs(next_height - current_height))
                heapq.heappush(minHeap, (next_diff, row, col-1))

            visited.add((row, col))